Interpreting Pi Squared: Led by Academic Elites from Top Universities
While BTC has been experiencing a downward trend over the past few days, the primary market has continued to reveal significant financing deals. Aside from the $85 million raised by the AI giant Sentiment, another noteworthy financing project this week is Pi Squared, related to ZK technology.
On July 2, Pi Squared announced that it had secured $12 million in seed funding led by Polychain Capital, with participation from ABCDE, Bloccelerate, Generative Ventures, Robot Ventures, and Samsung Next. Angel investors included Justin Drake from the Ethereum Foundation and Sreeram Kanaan, founder of EigenLayer.

From publicly available information, the name Pi Squared suggests profound mathematical implications, with the infinite nature of pi squared creating a sense of technical depth and mystery. The project itself focuses on building “universal ZK circuits” and a “universal settlement layer,” often described in a way that is hard to understand even though the words are familiar.
Despite the lofty and seemingly incomprehensible nature of the project, the combination of high-profile VCs and well-known individuals as angel investors makes Pi Squared difficult to ignore. So, what exactly is Pi Squared?
Pi Squared: Led by Academic Elites from Top Universities
Drawing Pi Squared on a blackboard might be baffling to many, but when academic elites do it, the effect is quite different.
Pi Squared is led by Grigore Rosu, a professor of computer science at the University of Illinois Urbana-Champaign (UIUC), who also serves as the project’s CEO.
In 2014, Grigore Rosu became a full professor at UIUC, specializing in formal verification and runtime verification. To put it simply, formal verification involves using mathematical methods to prove the correctness of software and hardware systems, ensuring they function properly under various conditions. Runtime verification, on the other hand, involves real-time monitoring of system behavior to enhance stability and security.
According to Wikipedia, Professor Grigore Rosu has published numerous papers that have received top awards from the Association for Computing Machinery (ACM) and the International Conference on Automated Software Engineering (ASE).
But what does this have to do with a crypto project?
If you look at the main business description of Pi Squared, you’ll find that it uses zero-knowledge proof (ZK) technology to verify the correct execution of any program, regardless of the programming language (PL) or virtual machine (VM) used. It provides a universal and efficient verification method aimed at achieving seamless interoperability between blockchains, languages, and applications.
This aligns perfectly with Professor Grigore Rosu’s expertise in verification, making it an ideal application of his academic knowledge.
USL: Facilitating System Transaction Settlement
Let’s take a closer look at the “universal settlement layer” (USL) that Pi Squared aims to create.
Firstly, it’s important to note that Pi Squared does not directly target end-users but rather provides products for various crypto and developer communities.
Pi Squared’s goal is to create a USL that simplifies interoperability between blockchains, languages, and applications, eliminating the need for traditional, often error-prone language implementations such as compilers, translators, or interpreters.
To understand it simply, you can think of it as a super translator that allows different blockchains, programming languages, and applications to operate seamlessly with one another. Developers can more easily create cross-chain and cross-language applications.
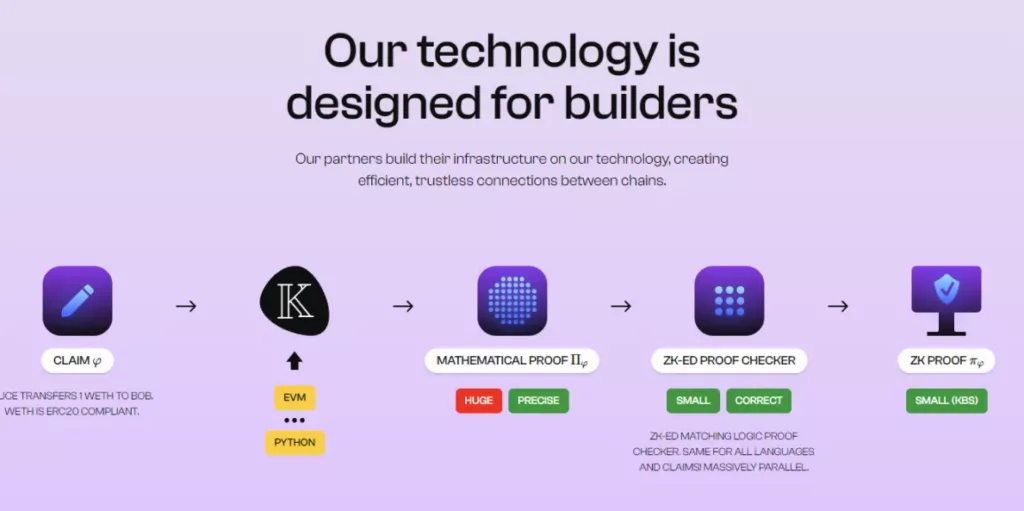
Here’s a diagram from the Pi Squared website illustrating this super translator’s role:
- Initiate Transaction: A user (e.g., Alice) initiates a transaction request, such as transferring 1 WETH to Bob. WETH is an ERC20-compliant token.
- Universal Settlement: The transaction request is submitted to an environment supporting any virtual machine and programming language, like EVM or Python. This is the universal settlement layer—computation can be expressed in any language or VM as long as there is a formal specification for that particular language or VM.
- Generate Proof: For step 2, a mathematical proof is generated to verify the transaction’s correctness. This proof is very precise but can be very large.
- Proof Verification: A ZK-ED (zero-knowledge – matching logic) proof checker is used to verify the “big proof.” This checker is small and can process large-scale parallel tasks.
- ZK Compression: Finally, a zero-knowledge proof is generated to verify the transaction’s correctness. This zero-knowledge proof is small and can be efficiently transmitted and verified.
Thus, the above process demonstrates how a simple transaction request can lead to the generation and verification of mathematical proofs, ultimately producing a smaller zero-knowledge proof to ensure the transaction’s correctness and privacy.
From the project’s ecosystem perspective, Pi Squared occupies a middle position in the technology stack, above the computation layer and sequencing services and below the consensus and data availability layer.
Pi Squared’s USL can be seen as an optimistic rollup that interprets and verifies the correctness of computational transactions, connecting the computation layer above with the consensus layer below.
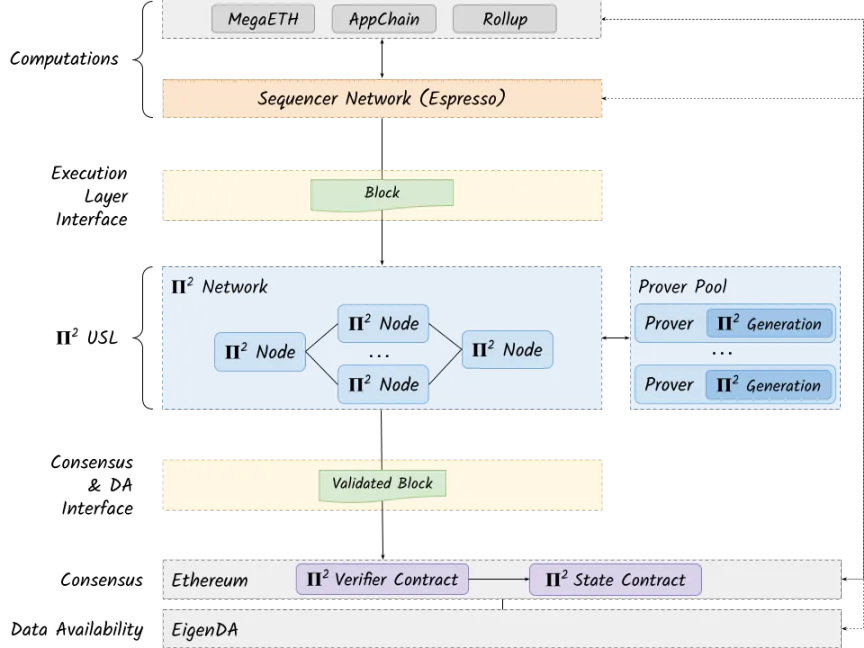
Moreover, Pi Squared mentioned having its own network with nodes running consensus protocols, suggesting a possible staking-based economic system directly related to the project’s token. However, no information about the token has been disclosed in the project documents so far, leaving room for speculation about its future.
Pi Squared is still in the proof-of-concept stage. Professor Rosu stated that the project would enter the testnet phase by the end of 2024. Currently, it is in the warming-up and promotional stage, with no activities or tasks available for participation.
We will continue to monitor the project. Regardless of any calls to avoid taking over, there will always be opportunities worth participating in.